not will it tow, but will it stop

[H20Rat]

Re: not will it tow, but will it stop

another overlooked thing that actually makes a HUGE difference, tires! Believe it or not, the more aggressive and off-road the tread pattern, the crappier it is at dry on-road panic braking. Given deep lug off road tires on a slightly heavier vehicle, and a good summer only tire on a somewhat lighter vehicle, the lighter one will outstop the heavier one with the same load hands down.

trick is to find the right compromise. if you don't actually truly go offroad, like the vast majority of 4x4's, buy a tire that better suits what you do drive on.

 

[45Auto]

Re: not will it tow, but will it stop

4,000lb vehicle + 3,000lb boat = 7,000lbs of resistance on four tires when braking slowly (1G)
4,000lb vehicle + 3,000lb boat = 21,000lbs (10 tons!) of resistance on those same four tires and brake pads when braking quickly (3Gs)

The real danger comes into play when you realize the latter example equates to 9,000lbs pushing on your vehicle while you are trying to stop.

Your intentions are good, but your physics are pretty much non-existent.

You're nowhere near even 1G of braking (MUCH less 3G !!!!) with any kind of tow vehicle. It takes a pretty high-end sports car to get to 1G of deceleration. A $100,000 Corvette ZR1 will BARELY do 1G in braking (it does 1.1 G). A normal street tire can get nowhere near enough traction to pull 1G.

1G will decelerate (or accelerate, it doesn't care which direction it's acting in) you at 22 MPH per second. So if you can stop your rig from 66 MPH in 3 seconds you're braking at 1G. If you can do it in 1 second, you're braking at 3G. Conversely, if you can accelerate from 0-66 MPH in 3 seconds, you're accelerating at 1G (The 638HP Corvette ZR1 does 0-60 in 3.3 seconds - not quite 1G).

The total vertical download on the tires remains the same (7000 lbs in your example) regardless of the deceleration. The DISTRIBUTION of that 7000 pounds is different from front to rear due to braking, but the total on the tires will ALWAYS be 7000 pounds. If you could brake hard enough so that your trailer tires and the rear wheels of your car came off the ground, your front tires would be seeing 7000 pounds. But you can't do that because the coefficient of friction between your tires and the pavement is not high enough.

Braking force available to a tire is determined by coefficient of friction times normal force. If your coefficient of friction was 1, your braking force could be equal to your normal force, which is 1G due to gravity on your tires. However, your tires may have a coefficient of friction of .8 if you're lucky. This means that the MAXIMUM braking force you could ever see is .8G.

If your example tow vehicle has 1000 pounds on each tire, braking force available on each tire will equal 1000 x .8 = 800 pounds. So once the braking force exceeds 800 pounds, the tire starts skidding. Once it's skidding, the coefficient of friction drops even lower (static friction is higher than kinetic (sliding) friction). That's why anti-lock brakes stop better, they don't let the tires skid and go into kinetic friction.

If your example 3000 pound trailer is a single axle trailer, each tire will have 1500 x .8 = 1200 pounds of braking force available. So the total braking force available to your example would be (4 x 800) + (2 x 1200) = 5600 pounds. You'll never see anywhere near 7000 pounds pushing on your tires, much less 21,000 pounds.

 

[bkwapisz]

Re: not will it tow, but will it stop

Being able to stop is important. But operating your rig at proper speeds is equally as important. All the brakes in the world will do you no good when you jack knife because you were over driving your rig when you had to slam on the brakes.

Too many people towing 3000 lbs boats driving 75 mph on the highway thinking they are the the "stuff" in their fancy new pickup. Just watch what happens when they have to panic stop that load. These folks scare me more than the 4 cylinder mini pickup towing at its capacity with no trailer brakes going 55 mph.

Epic importance. I can't tell you how many times I have seen over zealous drivers on the highway towing a trailer of some sort (usually a larger snowmobile trailer) who fly by us (we have no trailer) in a snowstorm in sub 30 degree temps in Northern Michigan. I cannot for the life of me understand what he's thinking, until about 4 miles up when I come upon the sea of brakelites because some dope is upside down in the ditch and his trailer load is strewn all over the place. Man, if you've got a trailer DO NOT PASS and slow yer self down.

 

[scoutabout]

Re: not will it tow, but will it stop

Add environmental factors like rain, and we're all pretty much riding a train that ain't gonna stop til it stops.

I recall getting cut off some years ago while towing in the rain (pre-antilock days). I thought I was being prudent cruising just below the speed limit in the right lane. I stomped the brakes and was rewarded with nothing but an eerie shhhhhhhhhhhh sound as I locked all the wheels up. Forward speed was not affected one iota and had the goon not kept on going across my lane and out of it to the off ramp he was aiming for I would have piled into him for sure.

As luck would have it the coast cleared just as the jacknife action began and I was able to get off the brakes and get everything straightend out again.

Real sobering lesson. That sound sticks with me to this day.

Drop your speed another 15 mph in the rain.

 

[kenmyfam]

Re: not will it tow, but will it stop

Too many people towing 3000 lbs boats driving 75 mph on the highway thinking they are the the "stuff" in their fancy new pickup. Just watch what happens when they have to panic stop that load. These folks scare me more than the 4 cylinder mini pickup towing at its capacity with no trailer brakes going 55 mph.

Completely agree. Worth taking a little longer to get to your destination and arriving safely.

 

[jkust]

Re: not will it tow, but will it stop

This discussion reminds me of the Winter Driving discussion here in Minnesota. We all know we need to drive slower on a snowy/icy road but many still don't. 4 wheel drive just ads to the overconfidence. Also someone mentioned that you don't need an HD truck to tow a 19 footer single axle trailer. Of course that is true but you need more than 3500 lb capacity. A heavily constructed/premium 19 footer is a lot heavier than you would think and generally holds a lot more gas than other 19 footers. Just the dry weight and trailer weight and you are well over 3500lbs. At the minimun a truck body is needed not to mention the mechanicals taking a beating on the tow vehicle.

 

[1970 Chieftain V]

Re: not will it tow, but will it stop

The law in California is trailer brakes on everything over 1500.

This is not true. Trailer brakes are not required on boat trailers under 3000LBS. The 1500 is applicable only to camp trailers and trailer coaches (5th wheel and toy hauler type).

 

[lowkee]

Re: not will it tow, but will it stop

Your intentions are good, but your physics are pretty much non-existent.

You'll never see anywhere near 7000 pounds pushing on your tires, much less 21,000 pounds.

Thank you pointing out the coefficient of street tires, 45auto, but you missed the point of the example. Braking coefficient calculations only change if the trailer is falling from the sky, not ramming you from behind, which is the case when towing. The values are momentum, not vertical force. In braking, increase in retardation G's would not increase the load on the tires unless you are on an incline. The 'added' weight would come as forward momentum.

Now, one might be tempted to say "Well, the trailer load gets pushed down on the hitch and therefore the back tires of the truck", which would be correct. The hitch (pun) comes in when you remember the trailer ball only gets ~15% of the load and of that transferred load, most of it works as lever/fulcrum action thereby lifting the front tires of the truck, lessening the braking effectiveness of those wheels. So in reality you have, at best, 10% of a trailer going towards added braking power and the other 90% just pushing you down the road.

So, to keep with my perfectly functional crude example, slowing a Geo Metro has little impact on the calculated mass of the vehicle, where slamming on the brakes essentially makes your Geo Metro a Buick until you either let off the brakes or come to a complete stop. Your brakes, tires and frame all take that added stress during that time and your stopping distance is increased. Add a trailer to the mix and the effect is increased further, especially since the trailer is pushing you rather than adding to overall vehicle weight (which would change the braking coefficient equation 45 pointed out).

The point of the example is the faster you try to stop, the more mass you are stopping.

 

[rbh]

Re: not will it tow, but will it stop

Here is a little story of what happened to me last week, I was pulling a cable trailer behind my bucket truck, bucket truck is about 20,0000 pounds empty, the cable trailers is about 3500 empty, we were running on a snow covered road, with wet snow coming down, and every time I would do an brake application the trailers brakes would lock up due to no weight/traction, so I dialed down the electric brakes to almost zero.
But here is the kicker,at 20-25 mph on a down hill 8 percent grade going into a corner, geared down and small brake applications the trailer slipped into the oncoming lane. The only way to keep the trailer behind the truck was to not give it any brake till after the corner.
The point, road conditions play a major part in how you drive and lots of expierence certainly helps.
rob

 

[PeterMcG]

Re: not will it tow, but will it stop

Let me start by stating that I am not the brightest guy with resepct to physics, so I may have missed something along the way. With that caveat in place, I hope you don't mind me going point by point so that I can try to be as clear as possible with my intended reply and points.

A crude example:
4,000lb vehicle + 3,000lb boat = 7,000lbs of resistance on four tires when braking slowly (1G)

A 1G deceleration is anything BUT slow for an car on the road. As 45auto pointed out, it takes a good sports car to reach a 1G deceleration.

4,000lb vehicle + 3,000lb boat = 21,000lbs (10 tons!) of resistance on those same four tires and brake pads when braking quickly (3Gs)

1. A standard car on the highway that is undergoing a 3G deceleration, is in the middle of a collision.
2. So people are clear, a "G-force" has nothing to do with weight or mass. It is a ratio of acceleration that a body is experiencing with respect to the acceleration that would result on that body as a result of gravity. A body that is acceleration at 43.874 mph per second is accelerating at twice the rate it would be accelerating due to gravity near the earth's surface (21.937 mph per second). If the two accelerations are ratio'd, you get a value of 2 -- hence the force resulting in the change in speed is sometimes called a 2G force or one might say that the body is experiencing a 2G acceleration.
3. I am still scratching my head trying to understand why you are indicating that 4k lb + 3k lb = 21k lb. <shrug>

Thank you pointing out the coefficient of street tires, 45auto, but you missed the point of the example. Braking coefficient calculations only change if the trailer is falling from the sky, not ramming you from behind, which is the case when towing.

Um, help me out....if a body is falling from the sky, I don't think the coefficient of kinetic OR static friction between the tire and the ground surface would apply. Not sure what you are trying to express.
If I understood 45auto correctly, he was indicating that the maximum braking force that the tires can apply is only a fraction of the normal force that the ground exerts against the tires.

The values are momentum, not vertical force.

I haven't found ANY units of momentum in your posts here....
If you are saying that vertical force has no bearing, then (to use your expression) "you missed the point" that 45auto was making about frictional force -- on level ground, frictional force has EVERYTHING to do with the vertical loading of the tires and thus determination of the maximal braking force that can be exerted before tire slip occurs.

The point of the example is the faster you try to stop, the more mass you are stopping.

So, you are saying that mass changes when undergoing a negative value acceleration (deceleration)?
This is....interesting.

 

[captharv]

Re: not will it tow, but will it stop

Ok, guys, break....

I have owned 14 boats, all trailerable in 40 years of boating.
In todays society, read--lawsuit crazy-- its very stupid (and unsafe) to operate a vehicle with a trailer which don't have the best brakes that can be had. If some other driver creates the situation, and you hit him, and your trailer brakes are faulty, you may be charged with a portion of blame.

One very important factor is that the surge brakes are very dependant on the tow vehicle brakes. If the towing brakes are not working, for whatever reason, then the surge brakes are not going to actuate.
I used to tow with a Dodge Ramcharger. It was tow rated at 7500# and had the factory H/D tow package. Driving down the road, pulling out of a ramp, etc all were excellent. I tow with an equalizer hitch. It is rigged to not impead brake avction, and actually helps braking by not letting the weight being transfered while braking to the trailer tongue, thus limiting the vehicle's front end lift. However, the brakes were crap
Dodge's problem was the Ram had disk front and drum rear. The front did all of the braking, since I could never get the rears to work right. Yes, I put in fatory spec'ed H/D shoes, replaced the wheel cylinders, and the master, changed out the "brake proportioner", tried everything. On wet pavement, I had to start stopping way ahead of time to avoid skidding.
I now have an Expedition 4X4 and the difference is night and day with its 4 wheel disks, and the ABS ststem. Even in panic stops. Even in wet weather. However, I drive slower than the speed limits. 55 on the highway, 30-35 in the city
The trailer is also 4 wheel disks, radial tires, and tortion suspension. In one panic stop, I got smoke out of the trailer tires, so it works.

BTW, in Florida, the specs for trailer brakes are:
"Any trailer over 3000# mus have brakes. If it has two axles, brakes must be on both axles. if you have 3 axles, only the front 2 must have brakes."
"The trailer and tow vehicle must stop within 50' at 20 MPH" ( Mine stops in 35). Note: test this in the corner of a shopping plaza, not on a street

To the poster who asked how to test surge brakes, read the last sentence above.

 

[45Auto]

Re: not will it tow, but will it stop

Not worth trying to teach basic physics here! At least PeterMcG understands!

I think Lowkee is using some terms a little differently than their common definition, and we're probably trying say the same thing.

The values are momentum, not vertical force.

Nope, the values are force (which is commonly measured in pounds). Since we're no where near lightspeed we don't have to worry about e=mc squared and the effects on mass.

In braking, increase in retardation G's would not increase the load on the tires unless you are on an incline. The 'added' weight would come as forward momentum.

You're wrong on both your basic statements here. Actually an incline DECREASES the load on your tires, because gravity is acting straight down. The download on your tires is the cosine of the angle of the incline times the force perpendicular to the road on your tires. That's why your brakes lock easier and it takes longer to stop going downhill. Since braking force equals tire coefficient of friction times force, you have less braking power available. For an extreme example, if your 7000 pound example were on a 90 degree incline (straight down!), the braking force available to you would be: Cosine of 90 degrees x weight of vehicle x coefficient of friction. Since the cosine of 90 is 0, that means you have 0 x 7000 x .8 = 0 pounds of braking force available to you if you're going straight down. In a more real world example, the cosine of 30 degrees is .87. On a 30 degree slope you would have half the braking force available to you that you do on flat ground.

The point of the example is the faster you try to stop, the more mass you are stopping.

You REALLY need to review the concepts of mass and momentum. Mass is a constant as PeterMcG pointed out, nothing you can do to change that.

Maybe we can simplify this if you understand momentum:

From Wikipedia:

You can ignore the calculus, and since we're going MUCH slower than the speed of light (186,000 miles per SECOND) see how it all comes down to the bottom line is F=ma (Force = mass times acceleration)?

Your 7000 lb rig has a mass of 7000/32.2 = 213 slugs. (Mass is a constant by the way, unless you're approaching light speed. Then we have to worry about e = mc squared).

From my earlier example, your tires exert a braking force of 7000 x .8 = 5600 pounds.

Plugging these numbers into the force equation gives: 5600 (Force) = 213 (mass) times a (acceleration).

Solving for acceleration gives 5600 / 213 = a, so a = 26.13 feet per second squared. So you are slowing down at 26.13 feet per second per second. In practical terms, it means that if you are doing 70 MPH, it will take you 3.94 seconds to reduce your velocity to 0 (your velocity is 70 x 1.467 = 103 feet per second, and you're knocking off 26.13 feet per second each second. 103/26.13 = 3.94).

To put it into G, we divide it by the acceleration of gravity, 32.2 feet per second squared: 26.13 / 32.2 = .8 G.

We can use the time to figure out how far it'll take to stop using the basic position equation:

X = (Initial position) + (Velocity x Time) + (Acceleration x time squared/2)

When we stop, the velocity will be 0. We'll call the point where we hit the brakes our initial x position (0), so the answer will be how far we go.

Plug the numbers in and get:

X = (0) + (103 * 0) + (26.13 x (3.94 x 3.94)/2) = 203 feet.

As you can see, momentum has nothing to do with any of the above. Weight and tire coeffiecient of friction are the only things that you need to know to determine stopping performance.

Now if we want to talk about thermodynamics and how hot your brakes are going to get, it'll probably be easiest to use momentum to calculate that ......

 

[lowkee]

Re: not will it tow, but will it stop

Not worth trying to teach basic physics here! At least PeterMcG understands!

I think Lowkee is using some terms a little differently than their common definition, and we're probably trying say the same thing.

Now, if you really thought that, your first post would be asking for clarity and not argumentative.

"your physics are pretty much non-existent."

Nope, the values are force (which is commonly measured in pounds). Since we're no where near lightspeed we don't have to worry about e=mc squared and the effects on mass.

In braking, increase in retardation G's would not increase the load on the tires unless you are on an incline.

You're wrong on both your basic statements here. Actually an incline DECREASES the load on your tires, because gravity is acting straight down.

<blah blah blah, tons of equations>

I'll put this in a simpler example which you may follow without your equations. I am after all, trying to teach, not impress.

You skid to a stop while going up hill.

Do you stop faster than stopping on level ground? Yes.

So, assuming we're still on the same page so far, where does the extra force come from which causes you to stop faster?

It comes from gravity; In this case the incline of the hill pressing against the tires as you stop the car. So, in review, your tires have more load while braking, and the faster you brake the more load you have on your tires.

Let's review what you said was opposite:

In braking, increase in retardation G's (faster braking) would not increase the load on the tires (opposing force) unless you are on an incline (an up hill).

You REALLY need to review the concepts of mass and momentum. Mass is a constant as PeterMcG pointed out, nothing you can do to change that.

Wow, my bad. I'll be sure to study up on the proper wording next time I explain a crude example to you. When I ignorantly stated you'll have more mass to stop (decelerate), I should have said momentum. Oh wait, mass * deceleration (stopping) is momentum. You handily quoted wikipedia on that one.

Maybe we can simplify this if you understand momentum:

From Wikipedia:
<more blah blah>

..it all comes down to the bottom line is F=ma (Force = mass times acceleration)?

Your 7000 lb rig has a mass of 7000/32.2 = 213 slugs.

Plugging these numbers into the force equation gives: 5600 (Force) = 213 (mass) times a (acceleration).

<more snip>

As you can see, momentum has nothing to do with any of the above. Weight and tire coefficient of friction are the only things that you need to know to determine stopping performance.

Where in your equation is the trailer? We are, after all, discussing trailer towing. Are you simply trying to impress people with your wikipedia skills or is there a followup equation where you mention the momentum of the trailer behind the vehicle? ..without using momentum, of course.

Here's an equation you can impress your friends with:

Your pissing contest <> learning.

In layman's terms, nobody learns anything from your pissing contest. That is the point here, right? To share knowledge rather than scare people away from it.

Did I state that in complex enough terms for you, or do you require bigger words?

 

[PeterMcG]

Re: not will it tow, but will it stop

Did I state that in complex enough terms for you, or do you require bigger words?

<shrug>
Maybe you should mention (again) that you have been beta testing Win7 since January.

 

[lowkee]

Re: not will it tow, but will it stop

<shrug>
Maybe you should mention (again) that you have been beta testing Win7 since January.

Wrong person, bud. Maybe you should mention your hooked on phonics didn't work out so well. Dr Bowtie said that, not me.

 

[PeterMcG]

Re: not will it tow, but will it stop

Wrong person, bud. Maybe you should mention your hooked on phonics didn't work out so well. Dr Bowtie said that, not me.

My apologies, you are right.
You mentioned that you are a M$ beta tester, you didn't mention that you beta tested Win 7.

Any help you can provide with learning the language would be appreciated (English is not my native tongue). I never did use the "hooked on phonics" -- it seems that one must be able to speak English before the program can assist with reading skills.

 

[45Auto]

Re: not will it tow, but will it stop

I am after all, trying to teach,

That's the only reason I'm bothering to reply to you. You're trying to teach that 2 + 2 = 7 (if you were at least close, say 2 + 2 = 5, I wouldn't worry about it). You?re trying to teach that it won?t hurt your impellor to run your boat without water. You?re trying to teach that the earth is flat. I'm trying to prevent people from getting bad information.

You skid to a stop while going up hill.

Do you stop faster than stopping on level ground? Yes.

You're good so far.

It comes from gravity

Still looking good!

So, assuming we're still on the same page so far, where does the extra force come from which causes you to stop faster?

Keep reading and you?ll learn.

It comes from gravity; In this case the incline of the hill pressing against the tires as you stop the car.

You're at least partly right. The decreased stopping distance in uphill braking does come from gravity, but it has nothing to do with the hill pressing against the tires. More slope = less load on tires. The decreased stopping distance comes from the fact that the sine component of the gravity vector is in the same direction as your deceleration vector. The tires actually have LESS load on them and less available braking force. An easy way to visualize it is to imagine the hill getting steeper and steeper. The steeper it gets, the less load you have on your tires. When it reaches 90 degrees, the load on your tires is zero (this is because the cosine of 90 degrees is 0, as explained in my earlier post).

As I stated in my earlier post, your braking force is the component of gravity perpendicular to the road times the tire coefficient of friction. So you can see that if you have NO component of gravity perpendicular to the road (on a straight up road, your gravity component would be parallel to the road) you have NO braking force available from the tires. It works the same way on lesser inclines. More slope = less tire loading (why do you think you make sure a scale is level before you weigh something? If you weighed your 7000 pound rig with a scale tilted at a 30 degree angle it would tell you it weighed 6090 pounds). Maybe the picture below will help:

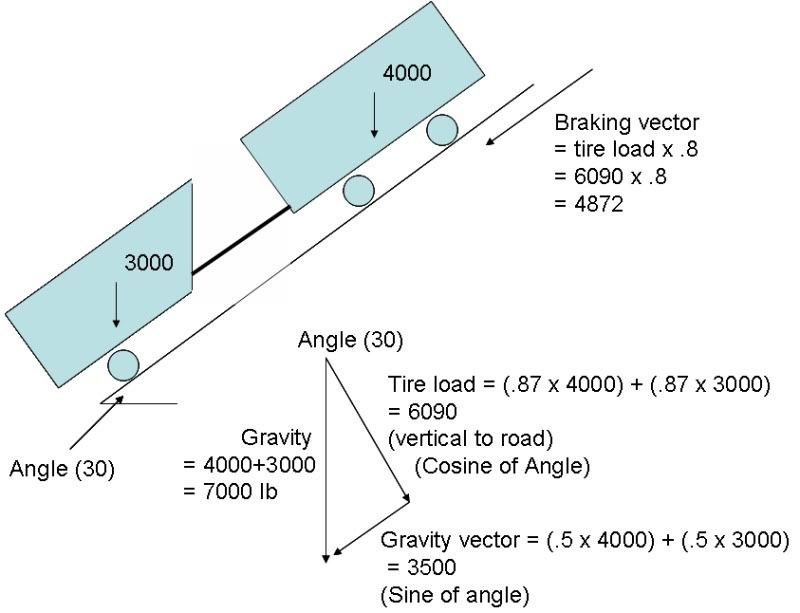
Your braking vector is opposite your direction of travel. If you?re traveling uphill, it?s in the same direction as the sine component of gravity. So in the example in the picture, traveling uphill, your total braking force is the sum of the force provided by your brakes (4872 lb) and the sine component (3500) of the gravitational force. Your total braking force would be 8372 pounds of force.

As stated in my earlier post, on flat ground, your braking force would be 5600 pounds.

If you were going downhill in the example picture the sine component of gravity would be in the opposite direction of your braking force (your braking force vector would point uphill). So you would have to subtract it from your braking force. Your braking force on a 30 degree downhill slope would be 4872 ? 3500 = 1372 pounds. If your braking force = 0 or less it means that the hill is so steep that your brakes won't hold you on it. You'll just slide to the bottom.

So the 3 cases we have with your 7000 pound example are:

Uphill (30 degree)= 8372 pounds of braking force = 8372/7000 = 1.20 G
Flat = 5600 pounds of braking force = 5600/7000 = .80 G
Downhill (30 degree) = 1372 pounds of braking force = 1372/7000 = .20 G

Oh wait, mass * deceleration (stopping) is momentum. You handily quoted wikipedia on that one.

WRONG, WRONG, WRONG!!

Your statement above pretty much sums up your whole problem. Read the Wikipedia post. If you do, you?ll see in the first sentence that momentum is mass times VELOCITY. It is NOT mass times ACCELERATION (or deceleration, same thing but opposite direction) as you stated above!! Apparently you do not understand the difference between velocity and acceleration and it is causing you to provide bad information.

Braking = Change in velocity = acceleration (deceleration is just acceleration with a negative direction)

MOMENTUM = mass x VELOCITY (velocity is not what we're talking about)

FORCE = mass x ACCELERATION (acceleration is what we're talking about)

If you're talking about braking, you're talking acceleration, NOT velocity. So you use force, NOT momentum. You're garbling stuff together that you don't understand and posting bad information.

Where in your equation is the trailer?

Not sure I understand what you?re asking. It's right there in the quote box you posted above your question:

Your 7000 lb rig has a mass of 7000/32.2 = 213 slugs.

Your example was a 4000 pound car and a 3000 pound trailer. Total is 7000 pounds that I used in all my equations.

Are you simply trying to impress people with your wikipedia skills or is there a followup equation where you mention the momentum of the trailer behind the vehicle?

If you would actually read the Wikipedia quote maybe you would understand how momentum is contained in the F = ma equation. It comes back to the difference between velocity and acceleration. You can't have acceleration without velocity. In calculus, you'll learn that Velocity is the first derivative of Position. Acceleration is the first derivative of Velocity, and the second derivative of Position. Velocity is an inherent component of acceleration. You can see it in the units:

Position = feet

Velocity = feet per second

Acceleration = feet per second per second

If you refuse to learn, no big deal. I'm just trying to keep other people from becoming confused by your misconceptions. Maybe if you could provide some references to your version of physics (good luck!) it would help. Here's you a link to a Physics 101 synopsis of braking:

http://www.physics.sc.edu/~rjones/phys101/braking.html

Count how many times "momentum" is mentioned .....

That is the point here, right? To share knowledge rather than scare people away from it.

I?m trying to share knowledge. Not sure what you?re doing.

 

[lowkee]

Re: not will it tow, but will it stop

Finishing this via PM

 

[Knightgang]

Re: not will it tow, but will it stop

Man, I really wanted to read the rest of this debate, as I think it is a good one from an educational point of view...

 

[lowkee]

Re: not will it tow, but will it stop

The summary is.. make sure you understand what you are disagreeing with before you attack someone. 45's examples all assumed the trailer had brakes installed (or was sitting on the roof of the car), my initial post was the opposite. All of the people jumping on the mob mentality bandwagon were making an oranges argument to an apples example.

So, after all of the bickering, a more accurate example could be made:

(4,000lb vehicle + 3,000lb trailer) * .2 Gs = 1,400lbs additional resistance to stopping your four tires must handle when braking slowly (stop light)
(4,000lb vehicle + 3,000lb trailer) * .6 Gs = 4,200lbs additional resistance to stopping your four tires must handle when braking quickly (accident avoidance)

You'll notice the only change is a reduced amount of G-force, going on what others have mentioned about the braking G limits before tire slippage on normal cars. The original example had 1 and 3 Gs, based on the only braking example I could find at the time.. a Formula One car braking limit of 5Gs. Hence, the 'crude example' many happily ignored and read as gospel.

The important factor which was ignored in the equations and insults in the debate is.. a trailer without brakes adds no addition braking to a tow vehicle, so it isn't like your car just weighs more. It is literally being pushed from behind whenever you let off the gas.